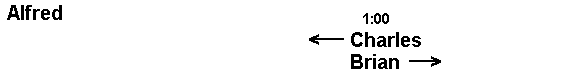for a fuller discussion and explanation of this.) It seems to me something like that might be happening with regard to interpretations of time in the typical physicists' explanations of relativity.
But first two points: 1) Bondi's argument (unless I am missing something) is fallacious. 2) Whether Bondi is correct or not, what he has written requires painstaking study by each individual's working through the details by him/herself. It took me more than a week, and numerous false or dead-end starts to arrive at, and see, what I am about to write, and I do not believe that is because I am stupid or slow; and Bondi is clearly not stupid or slow. One can be very brightly or cleverly mistaken, as I think Bondi is in this case. Bondi's writing and logic are so seemingly clear that (if I am correct) they make it that much more difficult to see the mistakes accidentally hidden within them.
I tried different examples, using my own math routes to "see" what was happening in the above Alfred/Brian/Charles situation. Each time, I came to an impasse because I could not generate the time discrepancies that Bondi gets. I was not even looking to disagree with Bondi's math; I was looking for a different interpretation of what the math signified, by applying, in an imaginary "thought-experiment" the principles he invoked, to phenomena other than light, so I could show that the interpretation he gave depended on what I thought would be a fallacy or mistaken presumption. But no matter what I did, I could not make the math come out to his answer in my own way of doing this. I couldn't get a time discrepancy between Alfred and Charles, so I went back to Bondi's explanation of the reciprocal differential interval ratios between velocities of approach and velocities of recession because I was not using that idea in trying to work through my own math. At that point I figured I was just misunderstanding the reciprocal principle of those time intervals. I was. But I think, so was Bondi.
Where the reciprocal notion comes about is the following from Bondi's book:
Imagine two observers, A and D, at a great distance from each other but who are not moving relative to each other. If A flashes a short pulse of light every 6 minutes toward D, then no matter how far away they are from each other, and no matter how long each beam of light takes to get to the other, they will still arrive 6 minutes apart (assuming the same path), even if this is 10 or 100 years later.
Now imagine a third observer, B, passing the first, on a path toward the second. Bondi sets B's relative and constant velocity such that, as the light pulses pass by him on their way to D, B observes them to be at an interval ratio of 3/2 to the six minute intervals they were transmitted because it will take progressively longer for each light pulse to catch up to B as he moves further and further from A. Hence, B will see these pulses sent out every 6 minutes by A's clock as getting to him every 9 minutes. There is an actual speed such that if B could travel it, this is what would happen. I will give an example of that later. Bondi then asks us to imagine that as these light pulses from A to D pass by B, B sends out his own light pulses toward D to accompany them. They will arrive at D at the same time each pulse of light from A does. Since A's pulses arrrive at D 6 minutes apart, so will B's pulses. But notice, B was sending out pulses 9 minutes apart on his clock, so that means that the arrival time intervals of the pulses are 2/3 of the sending time intervals. This is the reciprocal of the way the intervals worked from A to B. Bondi concludes from this, and I do not disagree, because I think that if you look at the math of this, that the approach interval ratio will always be the reciprocal of the receding interval ratio. And it is that fact he uses in the above argument regarding Alfred, Brian, and Charles that gets him to the discrepancies in time between the meetings of Alfred and Brian on the one hand and Alfred and Charles on the other hand depending on whether you are going by Alfred's watch alone or by the combination of Brian and Charles watches.
But the reciprocity of approach interval ratios and recession interval ratios will not do that, because, unless I am missing something, what those reciprocal ratios show has nothing to do with what Bondi thinks they show, and makes them seem to show. Mathematical calculations, even when accurate, do not always have the significance attributed to them. Let me first give two trivial examples of the misleading significance of correct math calculations:
1) Three guys go into a hotel and get a suite of rooms that cost $300 altogether. Each guy contributes $100 to the desk clerk. After they have gone to their rooms, the desk clerk realizes he made an error and the suite should have only been $250, so he gives the bellboy $50 to take back to the men. The bellboy does not know how to divide the $50 evenly among the men, so he instead gives each man $10, and he keeps the remaining $20 for himself. That means that essentially each man paid $90, which is $270 together. Take that $270 along with the bellboy's $20, and you have $290. But the original amount of money was $300, so what happened to the missing $10?
The answer is "nothing" because there is no missing $10. While it is true that $270 and $20 equal $290 and that $290 is $10 less than the original $300, those calculations have nothing to do with the situation. What needs to balance is the amount of money paid out and the amount of money taken in. The men paid out $270 ultimately. It is that money that needs to be accounted for, and it is easily accounted for: the desk clerk kept $250 of it and the bellboy kept $20 of it. You add the $20 to the $250 to get $270 (or subtract the $20 from the $270 to get $250). You do not add the $20 to the $270 because that is an insignificant calculation, giving an insignificant amount -- $20. If you want to deal with the original $300, that is accounted for by the $250 for the clerk, the $20 of the bellboy, and the $10 returned to each of the three men.
2) Suppose you go to the bank and deposit $100. Then one day you go in and withdraw $50, leaving you $50. On another day, you go in and you withdraw $25, leaving $25. Then you go in some time later and withdraw $10, leaving $15. You later withdraw $8, leaving $7 in the account. Then you withdraw $5.00, leaving $2.00, and finally you withdraw the last $2.00. Here is a table that shows all this activity:
|
Withdrawal |
Balance |
|
$50 |
$50 |
|
$25 |
$25 |
|
$10 |
$15 |
|
$ 8 |
$ 7 |
|
$ 5 |
$ 2 |
|
$ 2 |
0 |
But notice that when you add up the second column, it only comes out to $99. Where is the missing $1 from your original $100. If you add up the first column, it appears that you withdrew all $100, but clearly you didn't because there is a dollar missing from your balance. What is going on?
The answer is that nothing is going on with the money; just with the math. The problem has been constructed so that the balance column will add up to within a dollar of the withdrawal column, but the balance column is totally irrelevant to the initial deposit of the $100, as you can see if you took out $99 on the first withdrawal leaving a balance of $1, and then removed that $1 the next time. The balance column then would only add up to $1, yet the money would all be accounted for. Or if you instead took out $1 at a time from the original $100, leaving successive balances of $99, 98, 97, 96, ... which would add up to a great sum that obviously has no relationship to the sum of money involved. From the original presentation, it only looks like the sum of the balance column (which is perfectly good mathematics) has anything to do with the money in the sense that $1 seems to be missing.
In the Bondi presentation there is a similar psychologically misleading appearance, although the reciprocal interval ratios do have a real meaning and do represent something. It is just that what they represent has nothing to do with measuring time. I will explain shortly, but since this paper is meant to be as much about teaching and learning as it is about the math and physics of this particular case, I want say a little about how I arrived at these ideas while studying Bondi's book.
My first insight into this, after all the false starts, dead ends, and problems understanding the particulars when I tried to put it into my own words and perspective, came when I noticed that although Bondi was talking about light impulses, their actual speed had nothing to do with any of the mathematics. The mathematics only involves ratios, and those same ratios could apply to sailing ships at sea as well as they apply to space ships and light. Consider the following:
If there are two land masses a great distance apart, if ships traveling the same course at the same relative velocity set sail every six days from one port to the next, they will arrive at that second port six days apart, no matter how long the trip takes for each of them.
And, if at the same time the first ship sets sail, a slower ship towing many other ships, also sets sail, with a velocity that lets the six-day ships pass it every nine days apart as they catch up to it along the course, and if the towing ship sets loose one of the ships in tow that accompanies the passing 6-day ship, the ships the towing ship turns loose will arrive at 6-day intervals even though they were turned loose at 9-day intervals. Or instead of all these ships in tow, you can think of bottles with messages in them being tossed over to the six-day ships as they pass. The bottles, then with their messages, will arrive in six-day intervals with the ships, and thus the interval ratio will again be 6/9 or 2/3, since the bottles will be put into the ships every nine days and arrive every six days. Thus the reciprocity of the approaching/receding intervals holds even for ships moving relatively slowly. I will come back to this shortly to give a fuller explanation of what this means.
However, let us now apply the ship case to the Afred, Brian, Charles scenario given at the beginning of the paper, since nothing in that case has anything to do with specific fast velocities, but has to do only with relative velocities of Alfred, Brian, and Charles, no matter what those velocities are as long as they are related such that the approach/recession intervals are 2/3:3/2.
The diagrams are the same as above. For simplicity and to make this all easier to say, consider Alfred to be in a namesake port, from which Brian leaves on his way to a place that Charles is coming to Port Alfred from. Brian and Charles are moving at the same velocity toward each other on courses that let them pass close by each other. They each are towing lots of faster ships they can send on ahead (or, in Brian's case back) with messages. As Brian leaves Port Alfred, he notes the date for his log. Then, every sixth day, he sends back a ship to Port Alfred, and since Brian is receding from Port Alfred at a particular velocity established for this purpose, those ships will take 9 days to arrive back at the port, a 3/2 interval ratio. In 60 days, he passes Charles' ship, and Charles and Brian synchronize their calendars and the dates in their ships logs. Then every day after that Charles will set loose a ship as Brian had, with a message in it for Alfred.
The Bondi math calculation then will be this: In the first 60 days, Brian will have sent back 10 ships that arrive at Port Alfred every 9 days, so the last one will arrive 90 days after Brian has set sail. Then Charles' 10 ships, which he has set loose every 6 days, will arrive at Port Alfred every four days, for a total of 40 days. Hence, when Charles arrives at Port Alfred, by his and Brian's reckoning, 120 days will have passed, but by the calendar at Port Alfred, 130 days (90 + 40) will have passed. So time must change as one travels by ship at ship speeds.
But as far as I know, mariners and port authorities have never noticed or been plagued by this difficulty between the dates of sailing and arriving ships or passing ships -- because it does not happen and would not happen. It also does not happen if one merely mathematically, in a more straightforward fashion, calculates departure, arrival, recession and approach times, and launch/impulse-sending points along the way.
So something is wrong either with my understanding or with the with the reciprocal interval ratio math, or with how the math is being interpreted. It is the last that is the problem.
To show the mistake, imagine the following case:
There are two ports 3600 miles apart. Ships that travel 100 miles per day set sail every six days from Port Alfred to the other port, call it Port David. They will thus arrive six days apart at Port David (assuming the same course and constant velocity of 100 miles per day). Each ship will arrive in Port David 36 days after it sails from Port Alfred. The first one will arrive on day 36; the next on day 42; the third on day 48, etc. We will be able to calculate when the last one will arrive in a little while. First, however, Brian will set sail from Port Alfred to Port David at the same time as the first six-day interval ship sets sail for Port David. They start out side by side, but the six-day ship will be going faster. Brian will be sailing at a rate that lets each subsequent 6-day ship pass him 9 days after the previous ship passed him.
That means the first ship to pass him will pass him on day 9 of his journey, and since it was launched on the sixth day, it will take 3 days to catch up with him. This means that it traveled 300 miles to catch Brian, and since Brian had been traveling for 9 days to get that 300 miles, Brian's rate of sailing is at the rate of 33.3 miles per day. That means it will take Brian 108 days to reach Port David (3600 miles / 33.3 miles per day), and that is the day for which we will terminate our calculations. This will be 72 days after the first ship arrives, so there will be 72/6, or 12 six-day ships in all. When each ship passes Brian, he will toss the captain a message that says what day it is of his journey, and how many days it will be before he arrives at Port David.
So we can construct the following chart, given all this information:
(A)
Six-day
boat # |
(B)
Departure
Day of
Six-day
Ship |
(C)
Message
in Bottle:
Days From
My
Departure |
(D)
Message
in Bottle:
Days Until
My
Arrival |
(E)
Arrival
Day |
(F)
Days to Go
in Port David
Until Brian's Arrival
When This Ship
Arrives With the
Message |
|
1 |
0 |
0 |
108 |
36 |
72 |
|
2 |
6 |
9 |
99 |
42 |
66 |
|
3 |
12 |
18 |
90 |
48 |
60 |
|
4 |
18 |
27 |
81 |
54 |
54 |
|
5 |
24 |
36 |
72 |
60 |
48 |
|
6 |
30 |
45 |
63 |
66 |
42 |
|
7 |
36 |
54 |
54 |
72 |
36 |
|
8 |
42 |
63 |
45 |
78 |
30 |
|
9 |
48 |
72 |
36 |
84 |
24 |
|
10 |
54 |
81 |
27 |
90 |
18 |
|
11 |
60 |
90 |
18 |
96 |
12 |
|
12 |
66 |
99 |
9 |
102 |
6 |
|
13 |
72 |
108 |
0 |
108 |
0 |
Notice what the 3/2 and the reciprocal 2/3 interval ratios apply to: the 3/2 ratio is column C/B (after Brian's departure); and the 2/3 ratio is column F/D (before Brian's arrival). In other words, the 3/2 ratio applies to the interval between the six-day ship's departure day and its day of passing Brian; the 2/3 ratio applies to the interval between the six-day ship's arrival in Port David and how long it will be before Brian arrives, compared with how long it is between the ship's passing Brian and his arrival in Port David. The 3/2 interval ratio is simply reflective of the fact that each ship leaves Port Alfred 6 days after the previous one and catches up to Brian 9 days later: the catch-up interval is 3/2 of the leaving interval. But the 2/3 ratio is the ratio of the length of time between each ship's arrival and Brian's arrival, compared to the length of time between that ship's passing Brian and his arrival in Port David. Every time he tosses a message into the passing ship, for every day he has yet to go on his journey, it will be only 2/3 of a day between that ship's arrival in Port Charles and his own. The receding interval is a consequence of the distance Brian covers between each ships' leaving Port Alfred and catching him; the 2/3 approaching ratio is a consequence of how much Brian's ship closes on Port David as the ship that passed him beats him there. Since Brian is traveling at 1/3 the rate of the six-day ships, he covers 1/3 of the distance they do in the same amount of time, so when he is following a six-day ship from 300 miles out, he will get to port two days after it does, or 2/3 of the time later that it took. When he starts out ahead of a ship by 200 miles, they will meet 1.5 or 3/2 times further out in three days, because Brian will go that additional distance (100 miles) in the time it takes the ship to go the whole 300 miles.
As far as I can tell, neither ratio has anything to do with affecting calendar dates or clock times, whether we are talking intervals involving days, months, years, or micro-seconds or nano-seconds.
9) It is entirely possible that I have misunderstood or missed something in Bondi's argument so that it renders my whole exercise in #8 worthless from a mathematical or physics standpoint. It may also be that Bondi's characterization and explanation of relativity, if it is wrong, is simply flawed as a particular characterization and as a particular explanation of relativity, but that a better explanation of relativity may yield the same odd or "unintuitive" results, or results at odds with our narrowly conditioned understanding. Ino other words, Bondi's conclusion might be right even though his particular argument is faulty.
Nevertheless, I would like to use Bondi's book and my studying it to make some points about teaching and learning difficult material, perhaps particularly material that is logical or conceptual in nature.
a) One has to think while reading or listening to an explanation. No one can do the thinking for you, though they can help your thinking be more productive.
b) The reverse of the previous point is that while teachers can foster thinking or make it be more productive, they cannot do the thinking for students. Nor can they likely present material in such a way that students can understand it without thinking about it themselves.
c) Teachers can make material almost impossible to understand, or to want to understand, or to know it needs understanding, however, so there is a point to learning to teach well, in order to foster learning, even if one cannot automatically cause learning by one's teaching.
d) Teachers can teach students to work problems or repeat principles by rote, and that can make students masters of content in the sense that they can work problems and then teach others to do it, but that is different from their understanding the content themselves or being able to teach others how to understand the concept. The educational debate as to whether content understanding or teaching knowledge is more important in teaching is perhaps generally itself conceived improperly. If by having content knowledge, one means having the ability to apply the content successfully, that will not necessarily mean one can teach. But neither will that sort of knowledge along with pedagogical skills mean one can teach the subject either. Bondi's principle of reciprocal interval ratios is not difficult to apply, and for all I know it actually works to do give the same sorts of numerical results that the more typical relativistic calculations/transformations do. If so, I could have worked problems and taught others to work problems without going to all the effort I did to understand the explanation Bondi gave. But I could not have taught anyone to understand the concepts nor helped anyone work through the material until after I had studied it and discovered many of the things I described in #8.
In my article "The Concept and Teaching of Place-Value">, I make clear that there is a difference between being able to use place-value to add and subtract simple arithmetic -- as most arithmetic teachers and most adults can do -- and, on the other hand, being able to understand how the concept actually works to do what it does and what place-value really means conceptually. It is also my contention in that article that the reason students have so much trouble learning how to use place value is that it is taught by people who do not understand it and who cannot help students understand it, regardless of what pedagogical techniques they might know how to employ. There are some things you cannot teach if you do not know them yourself. Furthermore, I would contend that it is much more difficult to learn some things if you cannot make sense of them, and place value is one of those things. Students can learn to use place value in a day or two by understanding it, but it takes them much longer to learn to appy it correctly when they have to learn to apply it simply by repeated practice and drill. Moreover, you can learn the application from the understanding, but it is much more difficult to gain understanding from knowing the application, if you can do that at all. I believe relativity is another topic where this principle works also, as is much of physics and high school math. While it may be easy to teach students who do not need to be taught for understanding but who only need to be shown once or twice how to do an application, there are many more students who need to have understanding in order to be able to quickly learn to do the applications. Othherwise, they become lost and give up on a subject, mistakenly thinking they are just not smart enough to learn it or "no good at it". Yet they were only made to feel that way because they were taught by a teacher who did not understand the concept well enough to present it in a way that would facilitate learning by understanding. Even if that teacher was considered a content expert in terms of application, and even if that teacher was also considered a pedagogical expert in that they know what methodologies, if any, help different students learn various other things.
e) Finally, it is not clear to me what triggers puzzlement sometimes, what triggers misunderstanding, what triggers understanding of any given piece of material, such as Bondi's argument. I looked at and tried to work with his argument in many different ways even though I thought I understood it and accepted it. But I could not get things to come out in ways that made sense to me, and I had to keep re-reading it and re-interpreting it in my own words. Often I saw that my own words were not actually what Bondi was saying. Only then did I realize I was not sure what he was saying. It seems that it should have been easy to come up with the ship analogy, but it was not. At first, I tried to do it using sound because I wanted to show that since "sounds" of things were clearly not the "things" themselves, no matter what Bondi's argument might look like using speeds of sound, we would not accept the results about "time" and about the fastest things not aging, etc. But I could not get the math to work using sound, and I couldn't even get the argument to show up flawed using sound because I was still accepting using the reciprocal approach-recession interval ratios. I really got messed up trying to work the argument through using sound and the speed of sound. But it was at that point that I realized the argument did not involve high speeds at all, and I thought I could see it better if I dealt with more concrete phenomena such as the ships at sea. Still, I tried thinking it through either in my head, or with various abstract diagrams, including my own diagrams and those space-time diagrams Bondi has in the book, which look perfectly clear and perfectly intelligible. I just could not "see" what was wrong (or wrong with me) or what was puzzling me, nor could I see how these reciprocating ratios worked the way they did. For example, I was puzzled by why ratios had anything to do with it, and how you could somehow take something that began at six minutes intervals and then grew to nine minute intervals, and then shrink it back down to six minute intervals by subtracting three minutes of time that was somehow already past and therefore lost. I could see that if you multiplied the 9 by 2/3 you could get it back to 6, but I could not see why you knew to multiply instead of trying to subtract. Normally if you have to be somewhere in six minutes, and you become nine minutes late in the process, you cannot get there on time, no matter how well you multiply fractions.
So I knew I had to work out the step-by-step chart I finally ended up using in order to try to see what was going on, because I needed some sort of concrete, step-by-step example to work with. I also thought that the 2/3 approach ratio had to mean something, but I could not figure out what it meant -- what it applied to in physical reality. Even after I had the chart, with only some of the columns, I had to keep figuring out what columns or data I needed that might help, and I wasn't sure what they were. Even after I had the columns, it was not easy to see where the 2/3 relationship appeared. And even after I saw where it came from numerically, it was difficult to see what it signified or what it meant, or how it came about. As I was writing it and trying to explain it, I wrote some statements about what it meant that I decided were false, so I expunged them. Something that seems so simple when you read Bondi turns out not to be simple at all. One of the places where I really got confused, was in trying to account for where Charles was, and how to represent it, in sending out his first impulse transmission after his meeting with Brian. To Alfred it came four minutes later than Brian's last transmission, but I did not know how to show Alfred could tell that it was therefore transmitted closer to Alfred than further away. After all, from Alfred's point of view it was simply a later transmission that could have been sent by Brian 2 and 2/3 minutes after his previous transmission (on his clock), from further away, than to have been sent 6 minutes after the transmission by Charles from closer. And nothing that I could diagram seemed a satisfactory way of figuring out how to locate Charles' position at the point of transmission simply from knowing it arrived four minutes after the previous transmission. The receding transmissions and their intervals all made sense, but the approaching transmission intervals just did not make any sense to me, nor did they seem like they should to Alfred. One can understand one's perceptions in light of knowing the underlying phenomena, but it is difficult, if not impossible in some cases, to know the underlying phenomena or facts from just having the perceptions.
I hope that from all this it should be clear that teaching and learning complex conceptual and logical matters is not easy and that there are not likely to be any pedagogical tricks automatically known in advance for teaching or for learning such things. That said, I do believe that if you can figure out how to teach it to any kids who actually need instruction and who do not have an intuitive grasp of what is going on, that same approach will probably work for many students. Other than for things you learn to do by practice or rote, the art of learning is trying to make sense of what you don't know. The art of teaching is figuring out how to make sense of what you know and then figuring out how to help others make sense of it too.
)